

Projectile motion is the motion of an object thrown or projected into the air, subject only to acceleration as a result of gravity. The applications of projectile motion in physics and engineering are numerous. Some examples include meteors as they enter Earth’s atmosphere, fireworks, and the motion of any ball in sports. Such objects are called projectiles and their path is called a trajectory. The motion of falling objects as discussed in Motion Along a Straight Line is a simple one-dimensional type of projectile motion in which there is no horizontal movement. In this section, we consider two-dimensional projectile motion, and our treatment neglects the effects of air resistance.
The most important fact to remember here is that motions along perpendicular axes are independent and thus can be analyzed separately. We discussed this fact in Displacement and Velocity Vectors, where we saw that vertical and horizontal motions are independent. The key to analyzing two-dimensional projectile motion is to break it into two motions: one along the horizontal axis and the other along the vertical. (This choice of axes is the most sensible because acceleration resulting from gravity is vertical; thus, there is no acceleration along the horizontal axis when air resistance is negligible.) As is customary, we call the horizontal axis the x-axis and the vertical axis the y-axis. It is not required that we use this choice of axes; it is simply convenient in the case of gravitational acceleration. In other cases we may choose a different set of axes. Figure \(\PageIndex\) illustrates the notation for displacement, where we define \(\vec\) to be the total displacement, and \(\vec\) and \(\vec\) are its component vectors along the horizontal and vertical axes, respectively. The magnitudes of these vectors are s, x, and y.
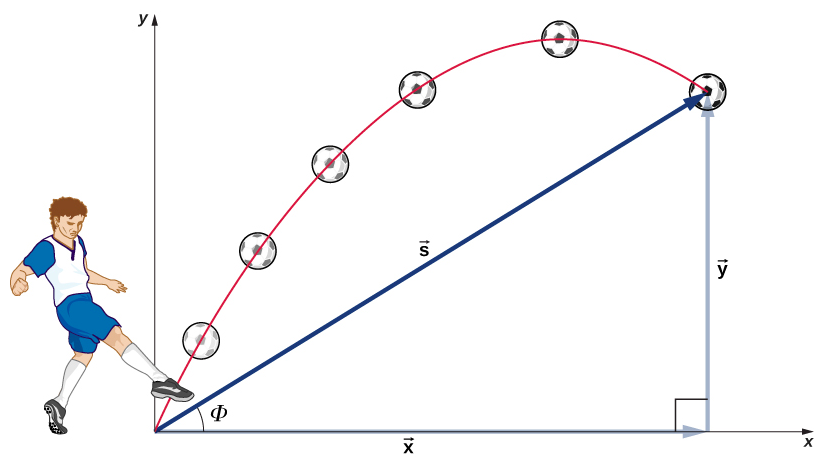
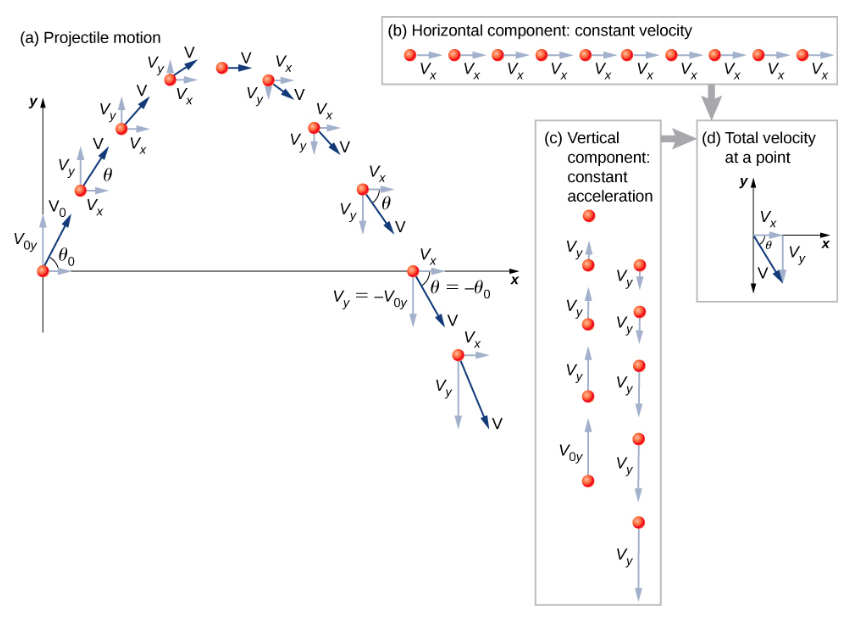
To describe projectile motion completely, we must include velocity and acceleration, as well as displacement. We must find their components along the x- and y-axes. Let’s assume all forces except gravity (such as air resistance and friction, for example) are negligible. Defining the positive direction to be upward, the components of acceleration are then very simple:
Because gravity is vertical, ax = 0. If ax = 0, this means the initial velocity in the x direction is equal to the final velocity in the x direction, or vx = v0x. With these conditions on acceleration and velocity, we can write the kinematic Equation 4.11 through Equation 4.18 for motion in a uniform gravitational field, including the rest of the kinematic equations for a constant acceleration from Motion with Constant Acceleration. The kinematic equations for motion in a uniform gravitational field become kinematic equations with ay = −g, ax = 0:
\[v_ = v_, \quad x = x_ + v_ t \label\]
Using this set of equations, we can analyze projectile motion, keeping in mind some important points.
During a fireworks display, a shell is shot into the air with an initial speed of 70.0 m/s at an angle of 75.0° above the horizontal, as illustrated in Figure \(\PageIndex\). The fuse is timed to ignite the shell just as it reaches its highest point above the ground. (a) Calculate the height at which the shell explodes. (b) How much time passes between the launch of the shell and the explosion? (c) What is the horizontal displacement of the shell when it explodes? (d) What is the total displacement from the point of launch to the highest point?
Strategy
The motion can be broken into horizontal and vertical motions in which ax = 0 and ay = −g. We can then define x0 and y0 to be zero and solve for the desired quantities.
A rock is thrown horizontally off a cliff 100.0 m high with a velocity of 15.0 m/s. (a) Define the origin of the coordinate system. (b) Which equation describes the horizontal motion? (c) Which equations describe the vertical motion? (d) What is the rock’s velocity at the point of impact?
A tennis player wins a match at Arthur Ashe stadium and hits a ball into the stands at 30 m/s and at an angle 45° above the horizontal (Figure \(\PageIndex\)). On its way down, the ball is caught by a spectator 10 m above the point where the ball was hit. (a) Calculate the time it takes the tennis ball to reach the spectator. (b) What are the magnitude and direction of the ball’s velocity at impact?
Strategy
Again, resolving this two-dimensional motion into two independent one-dimensional motions allows us to solve for the desired quantities. The time a projectile is in the air is governed by its vertical motion alone. Thus, we solve for t first. While the ball is rising and falling vertically, the horizontal motion continues at a constant velocity. This example asks for the final velocity. Thus, we recombine the vertical and horizontal results to obtain \(\vec\) at final time t, determined in the first part of the example.
Significance
Of interest are the time of flight, trajectory, and range for a projectile launched on a flat horizontal surface and impacting on the same surface. In this case, kinematic equations give useful expressions for these quantities, which are derived in the following sections.
We can solve for the time of flight of a projectile that is both launched and impacts on a flat horizontal surface by performing some manipulations of the kinematic equations. We note the position and displacement in y must be zero at launch and at impact on an even surface. Thus, we set the displacement in y equal to zero and find
\[y − y_ = v_ t − \frac gt^ = (v_ \sin \theta_)t − \frac gt^ = 0 \ldotp\]
Factoring, we have
\[t \left(v_ \sin \theta_ - \dfrac\right) = 0 \ldotp\]
Solving for t gives us
This is the time of flight for a projectile both launched and impacting on a flat horizontal surface. Equation \ref does not apply when the projectile lands at a different elevation than it was launched, as we saw in Example 4.8 of the tennis player hitting the ball into the stands. The other solution, t = 0, corresponds to the time at launch. The time of flight is linearly proportional to the initial velocity in the y direction and inversely proportional to g. Thus, on the Moon, where gravity is one-sixth that of Earth, a projectile launched with the same velocity as on Earth would be airborne six times as long.
The trajectory of a projectile can be found by eliminating the time variable t from the kinematic equations for arbitrary t and solving for y(x). We take x0 = y0 = 0 so the projectile is launched from the origin. The kinematic equation for x gives
\[x = v_t \Rightarrow t = \frac
Substituting the expression for t into the equation for the position y = (v0 sin \(\theta_\))t − \(\frac\) gt 2 gives
\[y = (v_ \sin \theta_) \left(\dfrac
Rearranging terms, we have
\[y = (\tan \theta_)x - \Big[ \frac <2(v_\cos \theta_)^> \Big] x^ \ldotp \label\]
This trajectory equation is of the form y = ax + bx 2 , which is an equation of a parabola with coefficients
\[a = \tan \theta_, \quad b = - \frac <2(v_\cos \theta_)^> \ldotp\]
From the trajectory equation we can also find the range, or the horizontal distance traveled by the projectile. Factoring Equation \ref, we have
\[y = x \Big[ \tan \theta_ - \frac <2(v_\cos \theta_)^> x \Big] \ldotp\]
The position y is zero for both the launch point and the impact point, since we are again considering only a flat horizontal surface. Setting y = 0 in this equation gives solutions x = 0, corresponding to the launch point, and
corresponding to the impact point. Using the trigonometric identity 2sin \(\theta\)cos\(\theta\) = sin2\(\theta\) and setting x = R for range, we find
Note particularly that Equation \ref is valid only for launch and impact on a horizontal surface. We see the range is directly proportional to the square of the initial speed v0 and sin 2 \(\theta_\), and it is inversely proportional to the acceleration of gravity. Thus, on the Moon, the range would be six times greater than on Earth for the same initial velocity. Furthermore, we see from the factor sin 2 \(\theta_\) that the range is maximum at 45°. These results are shown in Figure \(\PageIndex\). In (a) we see that the greater the initial velocity, the greater the range. In (b), we see that the range is maximum at 45°. This is true only for conditions neglecting air resistance. If air resistance is considered, the maximum angle is somewhat smaller. It is interesting that the same range is found for two initial launch angles that sum to 90°. The projectile launched with the smaller angle has a lower apex than the higher angle, but they both have the same range.
A golfer finds himself in two different situations on different holes. On the second hole he is 120 m from the green and wants to hit the ball 90 m and let it run onto the green. He angles the shot low to the ground at 30° to the horizontal to let the ball roll after impact. On the fourth hole he is 90 m from the green and wants to let the ball drop with a minimum amount of rolling after impact. Here, he angles the shot at 70° to the horizontal to minimize rolling after impact. Both shots are hit and impacted on a level surface. (a) What is the initial speed of the ball at the second hole? (b) What is the initial speed of the ball at the fourth hole? (c) Write the trajectory equation for both cases. (d) Graph the trajectories.
Strategy
We see that the range equation has the initial speed and angle, so we can solve for the initial speed for both (a) and (b). When we have the initial speed, we can use this value to write the trajectory equation.
Significance
The initial speed for the shot at 70° is greater than the initial speed of the shot at 30°. Note from Figure \(\PageIndex\) that two projectiles launched at the same speed but at different angles have the same range if the launch angles add to 90°. The launch angles in this example add to give a number greater than 90°. Thus, the shot at 70° has to have a greater launch speed to reach 90 m, otherwise it would land at a shorter distance.
If the two golf shots in Example 4.9 were launched at the same speed, which shot would have the greatest range?
When we speak of the range of a projectile on level ground, we assume R is very small compared with the circumference of Earth. If, however, the range is large, Earth curves away below the projectile and the acceleration resulting from gravity changes direction along the path. The range is larger than predicted by the range equation given earlier because the projectile has farther to fall than it would on level ground, as shown in Figure \(\PageIndex\), which is based on a drawing in Newton’s Principia. If the initial speed is great enough, the projectile goes into orbit. Earth’s surface drops 5 m every 8000 m. In 1 s an object falls 5 m without air resistance. Thus, if an object is given a horizontal velocity of 8000 m/s (or 18,000 mi/hr) near Earth’s surface, it will go into orbit around the planet because the surface continuously falls away from the object. This is roughly the speed of the Space Shuttle in a low Earth orbit when it was operational, or any satellite in a low Earth orbit. These and other aspects of orbital motion, such as Earth’s rotation, are covered in greater depth in Gravitation.
At PhET Explorations: Projectile Motion, learn about projectile motion in terms of the launch angle and initial velocity.
This page titled 4.4: Projectile Motion is shared under a CC BY license and was authored, remixed, and/or curated by OpenStax.